Advanced Institute deeply explores the specific application of empowering thermal interface materials

People often consult with advanced research institutes on how to apply the specific thermal pad flexibility data provided by manufacturers to actual systems. The flexibility of the thermal pad can be specified through deformation deflection and pressure curve. There has always been a misunderstanding about how the deflection deformation curve is generated and what useful information can be obtained from it. In some cases, this confusion may lead to the selection of poor thermal interface materials (TIMs). If you are familiar with the variables that affect the deflection deformation curve, you can understand their reactions in the system and optimize their efficiency, thereby improving heat conduction efficiency, saving costs, and increasing production efficiency. To understand how to achieve the above goals, it is best to start the discussion with thermal resistance.

Thermal interface materialTIM is an important component that provides a thermal conduction pathway for electronic components. These components wet and displace the air on the surface of the heating and cooling components, and fit with the tiny bumps and depressions on the mating surface. The wetting and heat transfer degree of TIM on the mating surface is quantified by the interface resistance (also known as "contact impedance"). This fit, combined with the high thermal conductivity of the thermal interface material, allows heat to quickly pass through the physical gaps between the components. Quantifying this overall heat transfer value is thermal resistance. For thermal conductivity system designers, the thermal resistance value of components is very important. They must ensure that the heat generated by the equipment can be properly dissipated to prevent overheating of the equipment.

The thermal resistance (Rth) of TIM in applications depends on three factors: the thermal conductivity of TIM (k), the thickness of the thermal interface material TIM in the application (t), and two interface impedances (Rint1 and Rint2) generated by the contact between the thermal interface material TIM and its two mating surfaces. The control equation for thermal resistance is as follows:

Rth= (t/ k) (Rint1 Rint2)

(Equation 1)

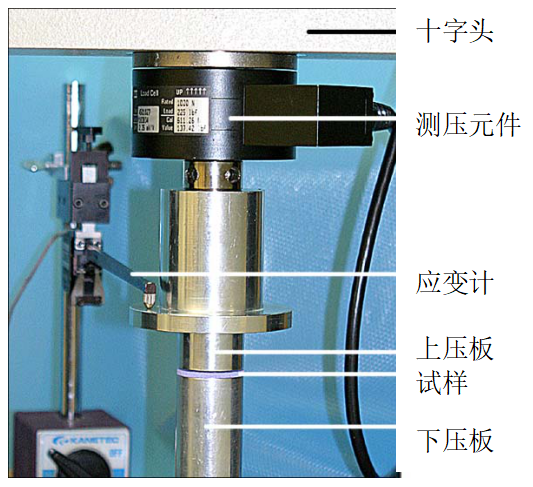
Generally speaking, the thermal conductivity of thermal interface material TIM is well known. However, if necessary, the coefficient can also be determined based on the relationship between thermal resistance and final thickness obtained from ASTM D5470. The thermal conductivity of the material is calculated based on the inverse slope of this line, measured in W/mK. The total interface resistance varies with the properties of the thermal interface material TIM and the contact surface, and therefore cannot be provided as a material property. However, the interface resistance of the system under test can be determined by the y-axis intercept on the same thermal resistance and final thickness relationship graph. If the thickness of the material is 0, then this point is the thermal resistance value of the material, and only the surface itself needs to be measured, thus simplifying equation 1 to Rth=(Rint1 Rint2).

The final thickness (t) of the thermal interface material TIM in applications is the main factor affecting the overall thermal resistance, especially for materials with medium to low thermal conductivity. For a specific system, the material thermal resistance of the heat channel is directly proportional to the distance over which heat must be transferred. To demonstrate this point, Figure 1 shows the effect of final thickness on total thermal resistance given thermal conductivity k and interface resistance of 0.97 cm2/W for three materials. This indicates that in order to efficiently transfer heat through the joint contact interface, it is necessary to minimize the thickness of the thermal interface material TIM as much as possible in the application. If the thermal conductivity Tc of the gasket is very low, this will be particularly important.